Resistor Basics
Resistors are Used For Lighting Up LEDs Smoothly
To turn ON an LED (Light Emitting Diode), the current flowing through the circuit must be limited to ensure that the voltage across the LED is at an appropriate level (around 2V). Consequently, connecting a single dry-cell battery (approx. 1.5V) directly as shown in Fig. 1-(a) will not emit light since the voltage is insufficient.
At the same time, connecting two batteries in series as in Fig. 1-(b) will result in overcurrent that destroys the LED since the voltage applied is too high (around 3V). But connecting a resistor between the dry cell batteries and LED as in Fig. 1-(c) allows the voltage applied to the LED to be controlled so that an appropriate current flows through the circuit, minimizing the amount of stress placed on the batteries and LED. In this way, a resistor provides the function of smoothing operation by limiting current flow, making it an essential component of electronic circuits.
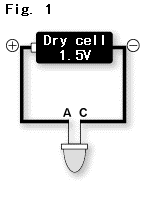
(a) No light emission
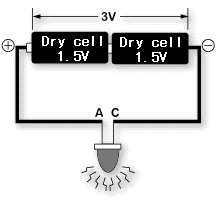
(b) Too much current flows
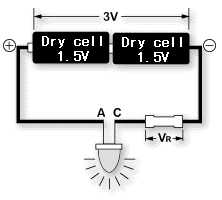
(c) Ideal operating condition
Recapture Ohm's Law
In the last example, it was mentioned that you need to use a resistor in order to operate an electric circuit, but let's take a closer look at how to determine the appropriate resistance value required in order to do so. This is given by the Ohm's law which states that the voltage(V) and the current(I) are related by the following equation:
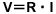
Here, R is the proportional constant of voltage(V) to current(I) and is called the electric resistance or simply resistance (unit: Ω). A resistor is simply this resistance realized as an electronic component.
What is the Size of the Resistor in Fig. 1 (c)?
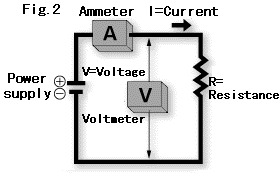
Let us now think of the meaning of Ohm's law. In the circuit of Fig. 2 above, if we assume that the voltage(V) is 1V and the current(I) is 1A, then according to the equation (1) above, the resistance(R) will be:
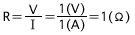
In other words, a 1(Ω) resistor is one through which a current of 1A flows when a voltage of 1V is applied across it. Or, the one which causes a voltage of 1V to appear across it when a current of 1A is passed through it. However, when the voltage remains the same at 1V but the current is 0.2A, the resistance value required is:
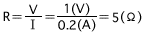
Also, the voltage required to pass a current of 1A through a 5(Ω); resistor is given by the following equation:
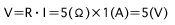
Thus, Ohm's law is always satisfied in an electric circuit, and hence if the values of any two of the voltage, current, and resistance are known, it is possible to determine the value of the remaining parameter.
Now, let's look at the value of the resistor required in the example of Fig. 1 (c). When the LED is operating normally, the voltage across its two terminals will be about 2V, and by subtracting the voltage of 2V across the two terminals of LED from the battery voltage of 3V, we get the voltage VR across the two terminals of the resistor as follows:
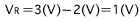
If we take the current ILED to be passed through the LED for normal operation as 15mA (1mA = 1/1000A), then the resistance(R) value required will be the following according to Ohm's law:

Therefore, the resistance value of the resistor in Fig. 1 (c) will be 67(Ω).
Power Consumption and Rated Power
Energy is required for people to be able to work, even electrical equipment such as motors, heaters, lamps, etc., require energy to operate. The amount of energy required Power consumption and rated power is expressed by the parameter called power consumption. Since electric power (even this power consumption) is expressed as a product of the voltage and current, the power P is given by the following equation:
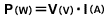
Since a voltage appears according to Ohm's law across the two terminals of a resistor when a current is passed through it, electric energy is consumed even in a resistor like electrical equipment. For example, when a current of 1(A) is passed through a resistor of 1(Ω), since a voltage of 1(V) appears across the resistor according to Ohm's law, the power consumption will be:
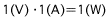
Thus the power consumption in the resistor is 1(W). In the case of a resistor, since this power will entirely be radiated as heat, if the power consumption is high, the temperature of the resistor itself increases, thereby finally burning it or melting it down. Therefore, it is necessary to indicate the amount of power the resistor can safely consume, and this power is called the rated power of the resistor. Normally, a resistor is used at a power consumption of less than 1/2 of the rated power so that we don't burn it out.
Serial Connection and Parallel Connection
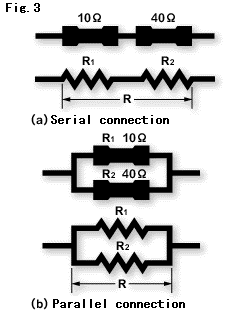
The connections in electric circuits can be classified broadly as serial connections and parallel connections which are shown in Fig. 3. When several resistors are connected together, the combined resistance value will be different in serial and parallel connections as follows:
In the case of serial connection:
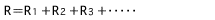
In the case of parallel connection:
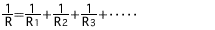
Consequently, the corresponding combined resistance values in Fig. 3 will be as follows:
(a) The combined resistance in the case of a serial connection is:
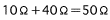
(b) The combined resistance in the case of a parallel connection is:
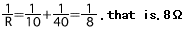
In other words, in the case of serial connection, the combined resistance value increases as the number of resistors in the connection becomes large and decreases in parallel connection as the number of resistors in the connection increases.
ROHM, the pioneer of chip resistors, offers a wide array of chip resistors that brings added value - in terms of greater reliability, increased miniaturization and improved performance - to sets of all types.